Figure 1.  A handy chart which has a variety of uses for telescope designers and makers.  The lines running from lower left to upper right represent the surface error, in wavelengths of light, of parabolic mirrors.  The intersection between these lines and the lines running from upper left to middle right indicate the focal ration at which mirrors may be left spherical.  Additional uses may be found by ambitious ATM's.

In this article we present a simple method of determining how far a "parabolic" mirror actually departs from parabolic figure, followed by a discussion on recommended tolerances.

The chart in Figure 1 shows the surface error on the mirror corresponds to a given measured departure from the desired knife-edge readings.  It works most conveniently with readings from a Foucault tester which measures knife-edge position in thousandths of an inch ( such as that described in Texereau's book* ).

Apparently a chart of this sort was first made in 1945 by Paul E. Luce, who had been a member of the optical division of the Amateur Astronomers Association of New York City.  I have modified his chart to make it more versatile.

HOW TO USE THE CHART:

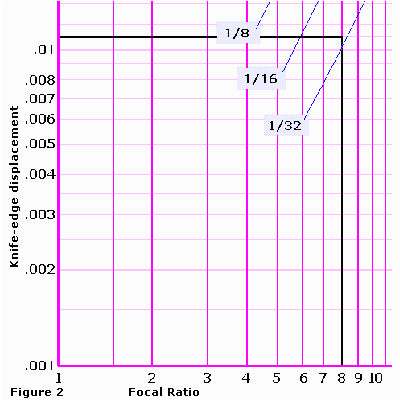
We make a set of knife-edge measurements for various zones of the mirror.  Beside these we tabulate the desired knife-edge positions ( r² / R ) for the measured zones.  To the measurements we add or subtract a constant so that the resulting numbers agree with the  r² / R  values most closely for the outer zones of the mirror  ( where a mirrors figure is most important ).  We then subtract these modified measurements from the r² / R values: The largest difference thus found ( which usually will be for the central zone of the mirror ) is what we call the maximum knife-edge error.  We draw a line across the chart from point representing the knife-edge error and note where this line intersects the vertical line representing the mirrors focal ratio.  Comparing this intersection point with the lines running diagonally from the lower left to the upper right tells us the maximum size of the mirror's surface error, in wavelengths of light.

This procedure is simpler than it may sound, as a couple of examples will make clear.  Suppose, for instance, that we test a 6 inch f/8 mirror, making careful knife-edge settings for the following four zones: r = .3 inch, 1.2 inch, 2.1 inch, 2.7 inch..  ( Note that the last zone is about 1/4 inch in from the mirror's edge, as the extreme edge is difficult to measure accurately. )  And suppose that we get the results listed in column C of Table 1.  The values of  r² / R for the four zones are listed in column D.

We find a constant ( in this case 0.124 inch ) which when subtracted from the measurements in column C gives numbers in column E which are nearly identical in zones 3 and 4 to the r² / R values.  Finally, column F lists the knife-edge errors ( column D minus column E ).  The largest residual, -0.011 inch is that for zone 1 as usual.  In Figure 2 I have drawn a heavy horizontal line at f8, the mirror's focal ratio.  These lines intersect very near the 1/32 wave chart line, indicating that the largest surface error on the mirror is approximately 1/32 wave.

Figure 2. Reproduction of part of Figure 1. Part of the chart has been omitted for greater clarity.  The heavy lines ( at 0.011 inch on the vertical axis and at f 8 on the horizontal axis ) represent the results of the reduction of a set of measurements for a 6 inch mirror, as described in the text.  The heavy lines intersect near the 1/32 wave chart line, which is thus the mirror's surface error.

As a second example, we test an 8 inch mirror of 40 inch focal length, using a Foucault tester adjusted to give a zero reading for the central zone.  Table II presents the results.  The constant in this case is 0.023.  The maximum knife-edge error turns out to be+0.025 inch.  Plotting this value on the chart with the mirror's focal ratio, f5, we find the surface error to be a bit less than 1/4 wave-- say 1/5 wave.

An easy way to find a constant which is to be added to or subtracted from the knife-edge reading is as follows:  for zone 3  subtract r² / R from the knife-edge reading; do the same for zone 4; the average of the numbers so obtained is the desired constant.  Thus for the 6 inch mirror of Table I, we have 0.172 - 0.046 = 0.126 and 0.198 - 0.076 = 0.122, so the constant is 0.124.  As a check on the arithmetic, when the procedure is followed correctly, the final knife-edge errors for zone 3 and 4 will be opposite in sign and approximately equal in size.

When the knife-edge error for zone 1 is a negative number, the mirror is under corrected ( a prolate spheroid, or a "partially parabolized" mirror ) ; when the zone 1 error is positive, the mirror is overcorrected ( a hyperboloid ).

A question often asked, especially by neophyte mirror makers who wisely would rather not rely upon uncertain judgments of Foucault shadows, is, "At what focal ratio may a mirror be left spherical and still perform satisfactorily?"  This question may be answered by referring to the chart lines drawn from upper left to middle right, which are plots of  r² / R for common mirror diameters.  The focal ratio at which a spherical mirror is within a given tolerance of a parabola is the focal ratio at which the mirror's r² / R line and the desired surface error line intersect.  Thus, for example, a 6 inch sphere is parabolic within 1/4 wave at f8.5 and a 4.25 inch sphere is within 1/16 wave surface error of a parabolic figure at f12, as the chart shows.

When may we consider a mirror finished?  Answering this involves answering three questions, as follows:

( 1 )  In the Foucault test, does the mirror present a good "doughnut" figure, with smoothly blending shadows?  Any irregularities in the figure should be polished out.

( 2 )  What about the mirror edge?  Usually this is the least satisfactory part of an amateur-made mirror, thanks to the cult of the perfectly figured edge which has been promulgated in all of the books on telescope making.  Turned edges ruin more Newtonian telescope images than any other cause except bad diagonals and metal telescope tubes.  Rare is the ATM who can produce a parabolic mirror with a perfectly figured edge.

But many ATM's don't know a defective edge when they see one, because the Foucault test is not adequate for detecting edge defects.  The mirror's edge must be tested either by a Ronchi test or by the knife-edge diffraction test described in section II-25 of Texereau's book.

In spite of what all the telescope making books say, the mirror need not have a good edge at the end of figuring.  There is no point in ruining the figure of the rest of the mirror in an attempt to correct a narrow turned edge.  But if the edge is not absolutely perfect, it must be either ground off of masked before the mirror is installed in the telescope.  Most professionals bevel the edge after figuring, thus grinding off edge defects.

( 3 )  Is the mirror's surface sufficiently close to the desired parabola. as determined, for example, by the chart introduced in this article or by the method described in Texereau's book?  Here, of course, we must first decide how close to parabolic figure is "sufficiently close".  The figuring tolerance depends critically upon what the telescope will be used for.  For the all-round observing in which the average amateur engages, the classic Rayleigh limit is an adequate tolerance.

Despite popular misconception, the Rayleigh limit does not refer to a 1/4 wave maximum surface error on the primary mirror.  Rather, it specifies a maximum "optical path difference" or "wavefront error" of one quarter wavelength of light.  For practical purposes we may say that the wavefront error is equal to 1 1/2 times the primary mirror's surface error.  Thus it turns out that a telescope consisting of a primary mirror which is parabolic within 1/10 wave and a diagonal mirror which is flat within 1/10 wave barely meets the Rayleigh criterion, since
1.5 ( 1/10 ) + ( 1/10 ) = 0.15 + 0.10 = 0.25 = 1/4 wave.

A telescope which is to be used extensively for observation of fine planetary detail should have a maximum wavefront error of 1/10 wave, which implies a 1/30 wave primary and a 1/20 wave diagonal.  Such instruments are rare, of course.  At the other end of the scale, a telescope with a maximum wavefront error of 1/2 wave ( e.g. a 1/4 wave primary and a 1/8 wave diagonal ) will fulfill the needs of many casual observers, as it will produce a sharp star image at low and medium magnifications and will provide pleasing views of the moon, wide double stars, Jupiter's moons and Saturn's rings, prominent star clusters, bright nebula and galaxies and so forth.

*Jean Texereau, How to Make a Telescope, Dover ( clothbound ); also available in cloth bound reprint.

BACK